
Just the orange points has shifted to the right by two. Onto one of the vertices, and notice I've now shifted Let's translate, let's translate this, and I can do it by grabbing That same direction, and I'm using the Khan Academy To show you is a translation, which just means moving all the points in the same direction, and the same amount in Transformation to this, and the first one I'm going This right over here, the point X equals 0, y equals negative four, this is a point on the quadrilateral. You could argue there's an infinite, or there are an infinite number of points along this quadrilateral. Of the quadrilateral, but all the points along the sides too. Not just the four points that represent the vertices

For example, this right over here, this is a quadrilateral we've plotted it on the coordinate plane. It's talking about taking a set of coordinates or a set of points, and then changing themĭifferent set of points. You're taking something mathematical and you're changing it into something else mathematical, In a mathematical context? Well, it could mean that Something is changing, it's transforming from Transformation in mathematics, and you're probably used to Make your own mind maps with Mindomo.Introduce you to in this video is the notion of a It was created using Mindomo mind mapping software. If you need a review of the definitions of polygons (regular, irregular),press the button polygons and you can find a review. Renditions can also reproduce most original patterns. Tessellated floors are formed from tiles of varying size, shape and colour that fit together to produce a strongly geometric pattern that is repetitive.Suitable for heritage & traditional settings, simple patterns can also be created for use in more modern and contemporary styles. Aged 68, he stated, "Filling two-dimensional planes has become a real mania to which I have become addicted and from which I sometimes find it hard to tear myself away." Here is a very shortened version of how this came about. During his life, he became obsessed with filling surfaces with pictures that did not overlap or leave spaces. He is famous for his impossible depictions like the one on the right, but he was a master of lino and wood cuts and produced many superbly crafted landscapes as well. Can you locate it?Įscher can be regarded as the 'Father' of modern tessellations.

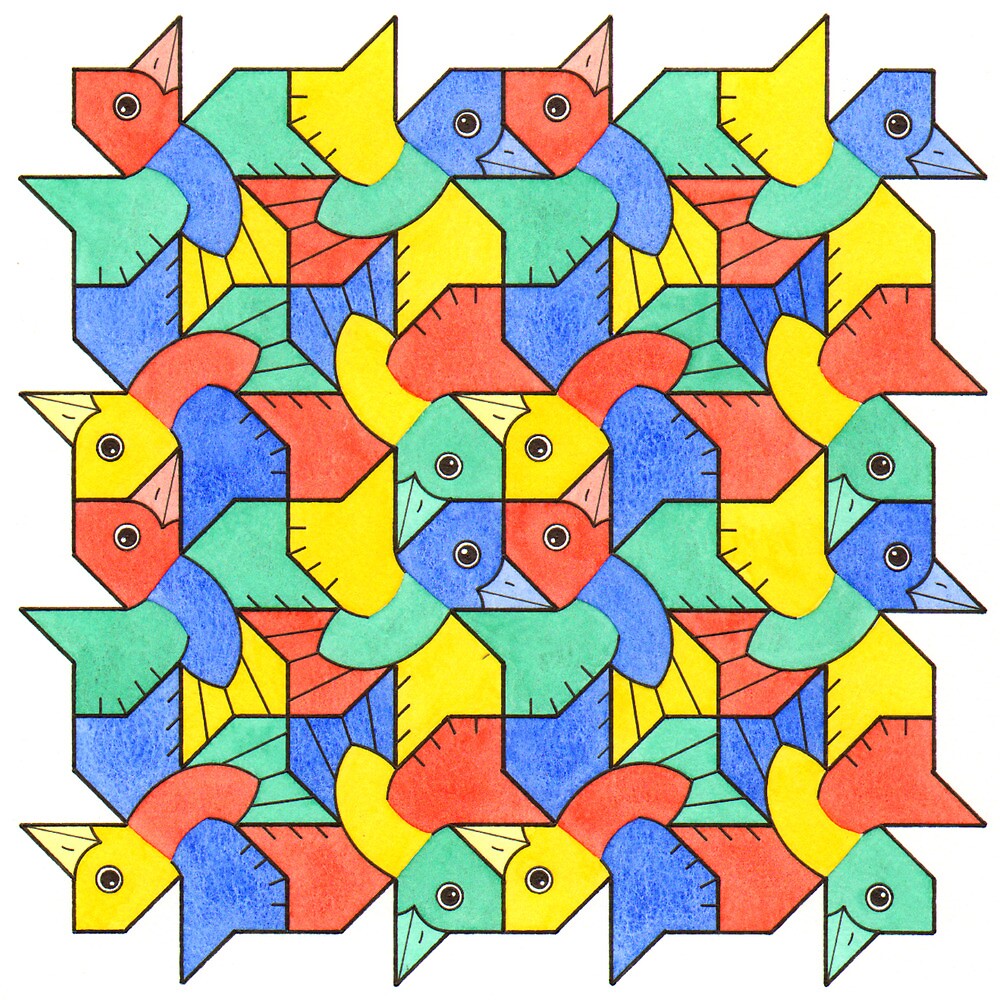
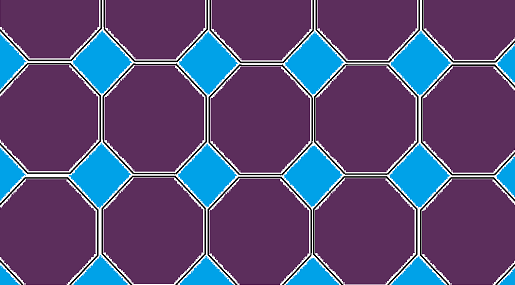
It marks the midpoint of a side of the parent triangle. There is also a point of 2-fold rotation on each fish's contour. Joining these points on the same fish in cyclic order will outline an equilateral triangle. Each of these points marks a location at which more than two fish meet. Ignoring color differences, there are centers of 6-fold rotation at the upper tip of each fish's right wing. There are centers of 3-fold rotation at the upper tip of each flying fish's left wing and the left tip of its tail. This tessellation has both translational and rotational symmetry. It is interesting to see basic geometric ideas and drawing techniques which Escher did by creating tessellations. It is interesting to study his art of tessellations and compare his tessellation to geometric forms. Related to this topic is the work of the artist M.C. If you need a detailed description of that principles, push the button. There are basically 4 ways of how a diagram can be “mapped onto” itself, namely, by translation, rotation, reflection and glide reflection.We will discover in the following diagrams, how this design can be “mapped onto” itself, which is the fundamental idea of how tessellations work. To illustrate the principles behind a simple tessellation pattern, a tiling consisting of equilateral triangles of degree 6 at each vertex will be used as an example to illustrate these principles. There are certain principles of tessellations. There are exactly three regular tessellations composed of regular polygons symmetrically tiling the plane. Tessellations however, do not need the use of regular polygons, below is an example. By definition, tilings require the use of regular polygons put together such that it completely covers the plane without overlapping or leaving gaps. There is a difference between a tiling and a tessellation. In other words a tiling of regular polygons (in two dimensions), polyhedra (three dimensions), or polytopes (n dimensions) is called a tessellation. What are tessellations? It is an arrangement of closed shapes that completely cover the plane without overlapping or leaving gaps.


 0 kommentar(er)
0 kommentar(er)
